
Sia

Question
Math
Posted 8 months ago
marks] Let be a vector space and let be a basis.
a) Define what it means that the triple is the coordinate vector of with respect to the basis .
Page 1 of 2
School of Mathematics and Statistics
University of New South Wales
b) Find the coordinate vector of with respect to the basis .
c) Define what it means that a matrix is the matrix of the linear map with respect to the basis .
d) Let
be the matrix of the map with respect to the basis . Find in terms of .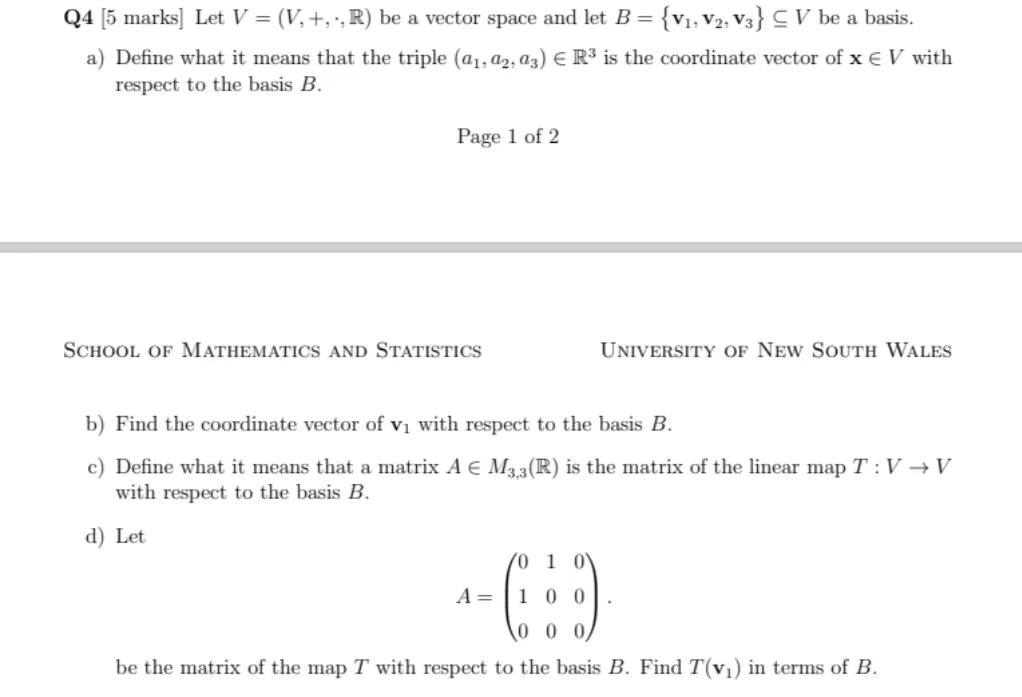

Answer from Sia
Posted 8 months ago
Solution by Steps
step 1
The coordinate vector of with respect to the basis is a unique ordered triple such that
step 2
Part (b)step 1
The coordinate vector of with respect to the basis is found by expressing as a linear combination of the basis vectors
step 2
Since is itself a basis vector, its coordinate vector is
Part (c)step 1
A matrix is the matrix of the linear map with respect to the basis if
step 2
is the matrix whose columns are the coordinate vectors of with respect to
Part (d)step 1
Given the matrix , we need to find in terms of
step 2
The first column of represents the coordinate vector of with respect to
step 3
Therefore,
Answer
Key Concept
Coordinate Vector
Explanation
The coordinate vector of a vector with respect to a basis is the unique ordered triple that expresses as a linear combination of the basis vectors.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question