
Sia

Question
Math
Posted 9 months ago
We want to find . Direct substitution and other algebraic methods don't seem to work.
Looking at the graph of , we can estimate that the limit is equal to 1 .
To prove that , we can use the squeeze theorem.
Pablo suggested that we use the functions and in order to apply the squeeze theorem.
Does Pablo's suggestion seem to be correct?
Choose 1 answer:
(A) Yes, Pablo's suggestion seems to be correct.
(B) No, Pablo's suggestion is incorrect because it's not true that one function is always below and one function is always above it for -values near 0 .
(c) No, Pablo's suggestion is incorrect because it's not true that the limits of and are both equal to 1 .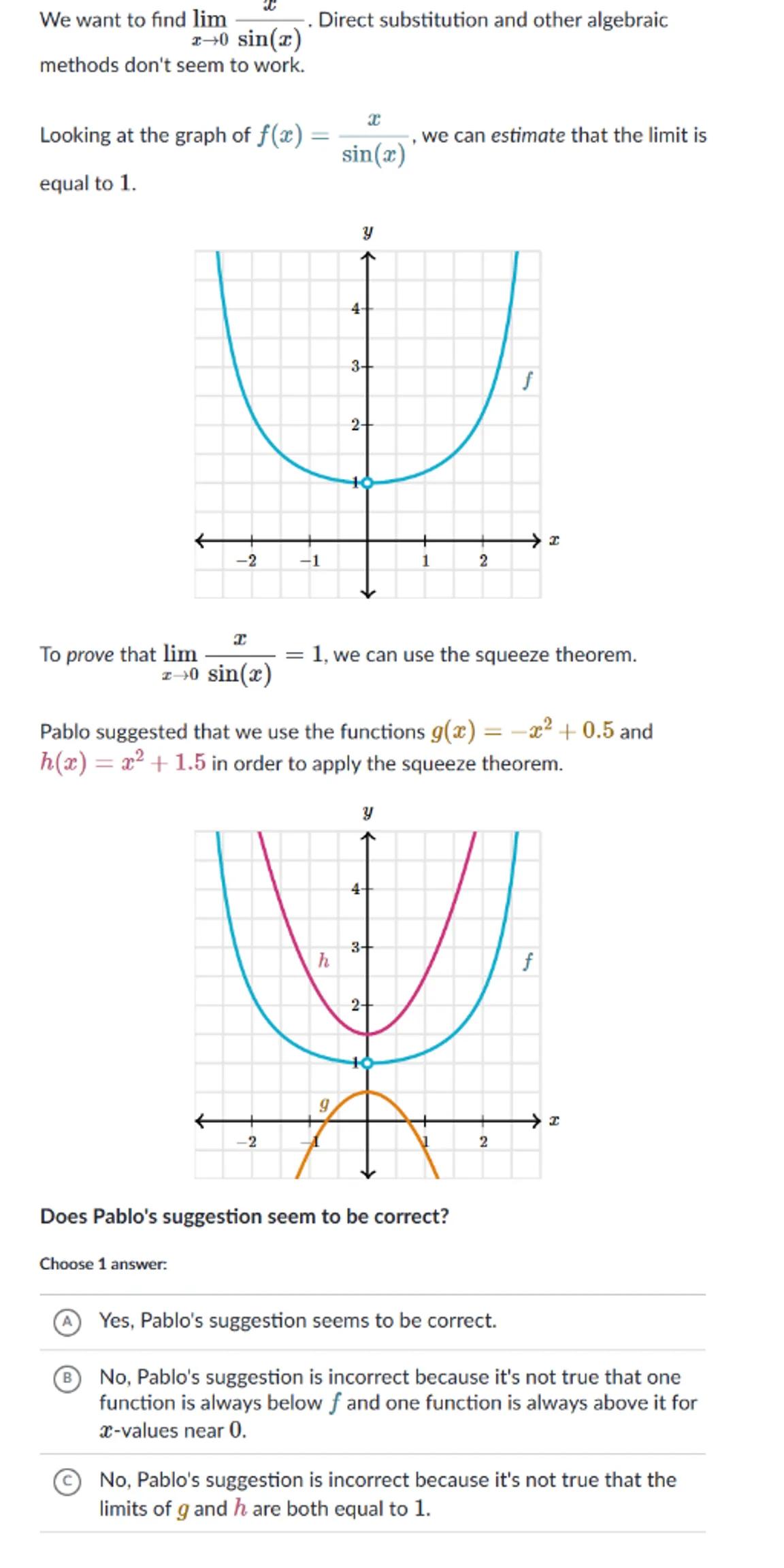

Answer from Sia
Posted 9 months ago
Solution by Steps
step 2
Looking at the graph of , we can estimate that the limit is equal to 1
step 3
To prove that , we can use the squeeze theorem
step 4
Pablo suggested that we use the functions and in order to apply the squeeze theorem
step 5
The limits of and as approaches 0 are and
step 6
Since the limits of and are not both equal to 1, Pablo's suggestion is incorrect
C
Key Concept
Squeeze Theorem
Explanation
The squeeze theorem requires that the limits of the bounding functions be equal to the limit of the function in question. Since the limits of and are not both equal to 1, Pablo's suggestion is incorrect.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question