
Sia

Question
Math
Posted 10 months ago
The theater director offered every member of the drama one vote for which play they preferred to perform. The director found that voted for The Oddems Family, that voted for Thirteenth Night, and that did not vote.
In this group, are the events "Oddems Family" and "Thirteenth Night" mutually exclusive?
Choose 1 answer:
(A) Yes
(B) No
Find the probability that a randomly selected person from this group voted for Oddems Family OR Thirteenth Night.
Oddems Family OR Thirteenth Night 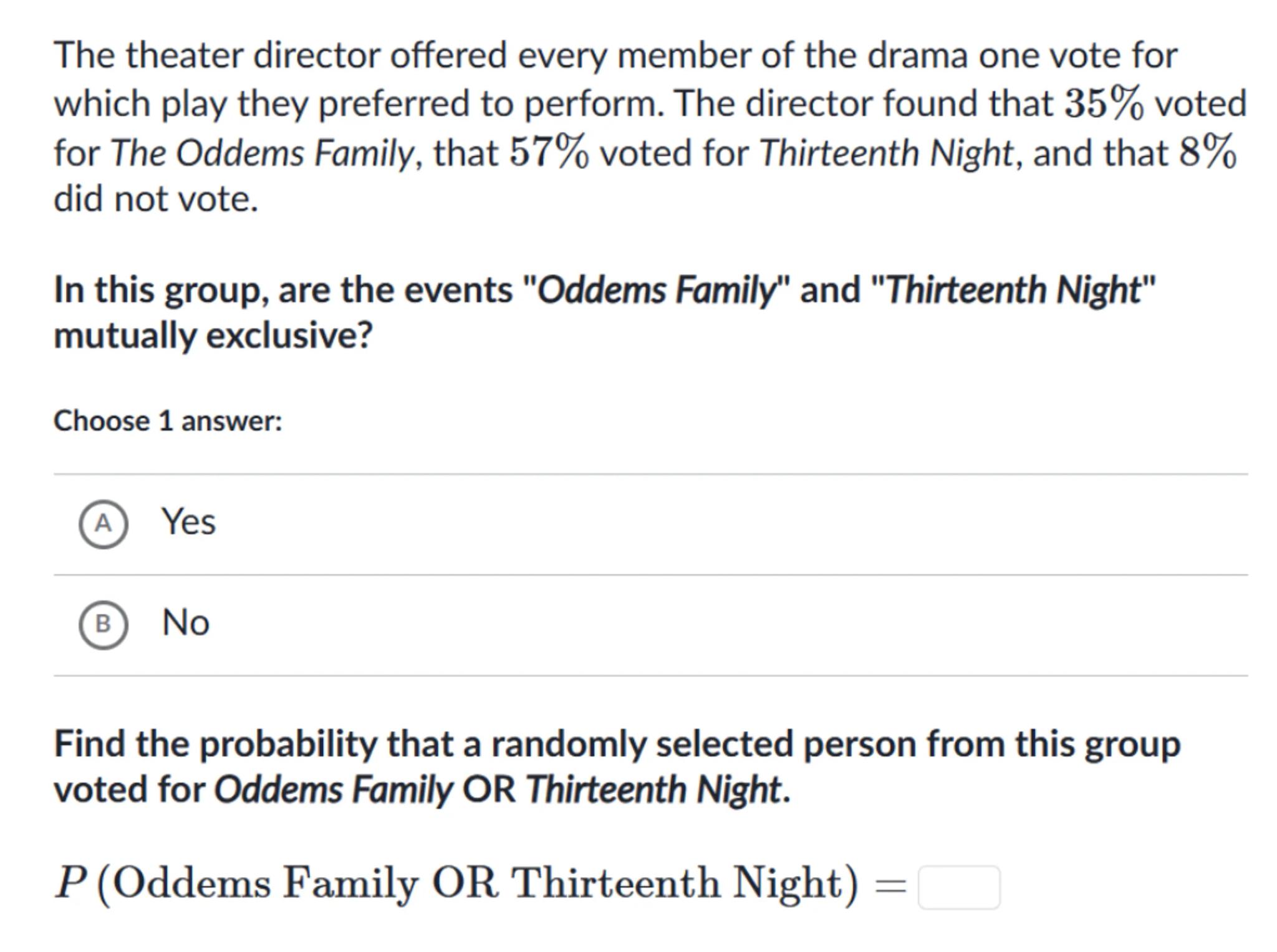

Answer from Sia
Posted 10 months ago
Solution by Steps
step 2
Since each member of the drama group is given only one vote, they can either vote for "Oddems Family," "Thirteenth Night," or not vote at all
step 3
Therefore, a member cannot vote for both "Oddems Family" and "Thirteenth Night" at the same time, making these events mutually exclusive
step 4
To find the probability that a randomly selected person voted for either "Oddems Family" OR "Thirteenth Night," we add the probabilities of each event since they are mutually exclusive
step 5
The probability of voting for "Oddems Family" is and for "Thirteenth Night" is
step 6
Adding these probabilities gives
step 7
Therefore, the probability that a randomly selected person voted for either play is or in decimal form
[1] Answer
A
[2] Answer
Key Concept
Mutually Exclusive Events
Explanation
Two events are mutually exclusive if they cannot occur at the same time. In this scenario, since each member can only cast one vote, they cannot vote for both plays, making the events mutually exclusive.
Key Concept
Probability of Union of Mutually Exclusive Events
Explanation
The probability of the union of two mutually exclusive events is the sum of their individual probabilities. This is because the occurrence of one event does not affect the occurrence of the other.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question