
Sia

Question
Math
Posted 10 months ago
Segment is an altitude of .
The figure is not to scale.
Which of the following additional statements would allow us to prove that ?
Choose 1 answer:
(A) only
(B) only
(C) Either statement is sufficient.
(D) We need both statements.
(E) Even with both statements, we still could not prove that .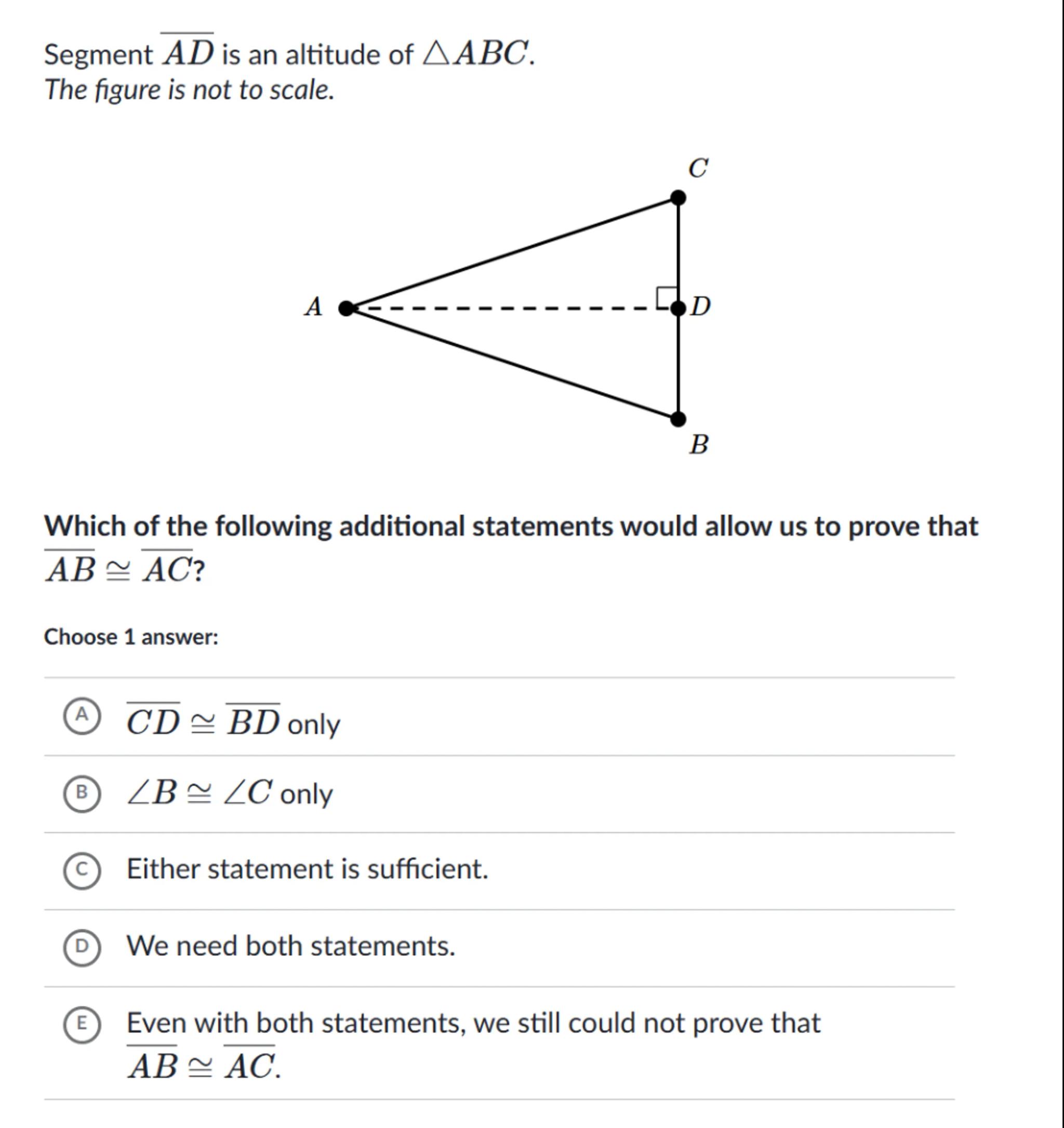

Answer from Sia
Posted 10 months ago
Solution by Steps
step 2
If , then by the Hypotenuse-Leg (HL) congruence theorem for right triangles, , since is a common side and is the altitude, making the perpendicular leg
step 3
If , then by the Angle-Side-Angle (ASA) congruence theorem, , since is a common side and is the altitude, and is the base for both triangles
step 4
Therefore, either statement (A) or (B) alone is sufficient to prove that
[question number] Answer
C
Key Concept
Congruence of Triangles
Explanation
To prove two triangles congruent, we can use congruence theorems such as HL for right triangles or ASA for any triangles, given the appropriate congruent parts.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question