
Sia

Question
Math
Posted 10 months ago
Let be a 2D vector field.
Is the expression a scalar field, a vector field, or undefined?
Choose 1 answer:
(A) Scalar field
(B) Vector field
(C) Undefined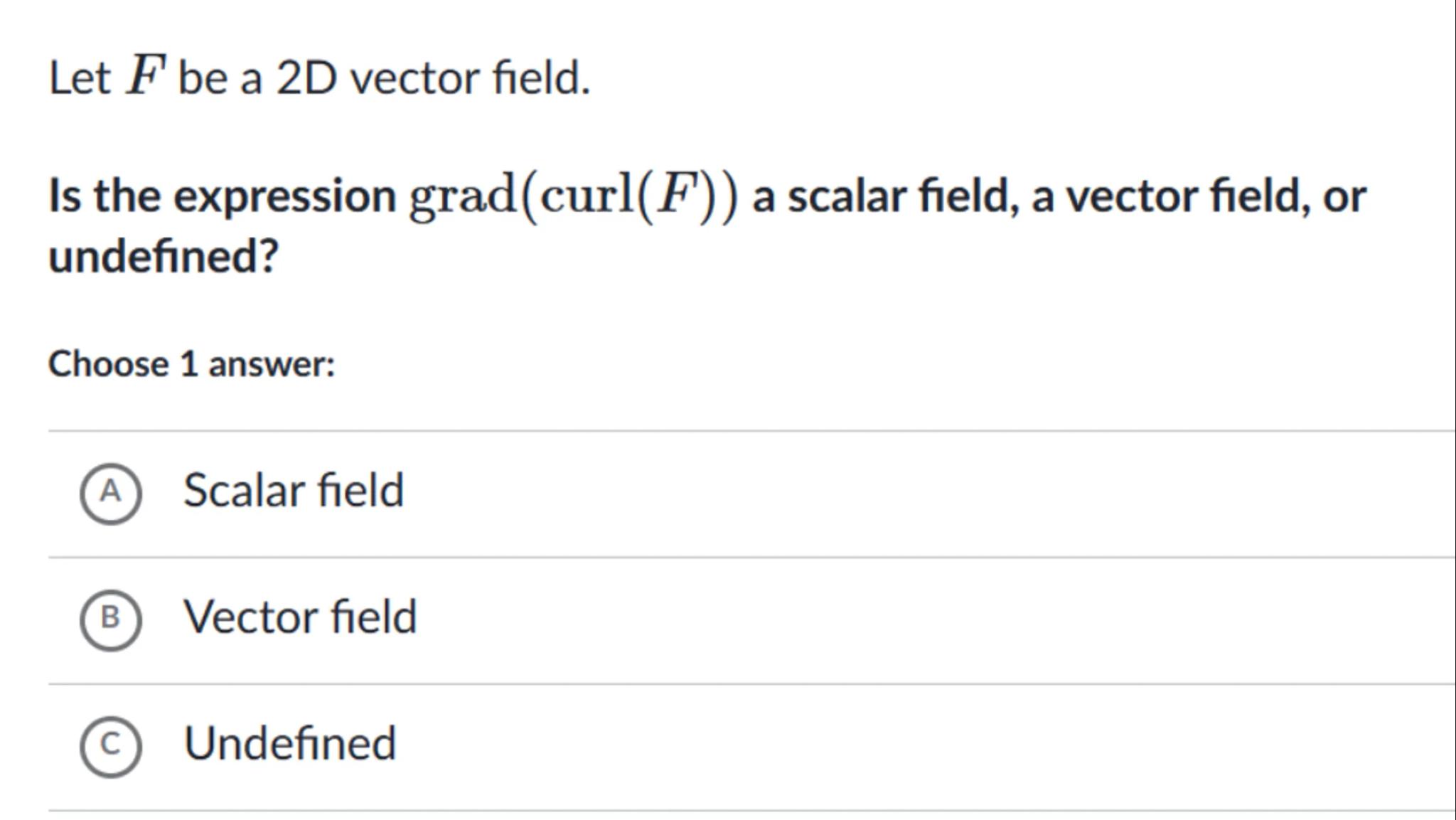

Answer from Sia
Posted 10 months ago
Solution by Steps
step 2
The curl of a 2D vector field is a scalar function, not a vector
step 3
The gradient (denoted as ) of a scalar function is a vector field
step 4
Therefore, the expression takes the curl of , which is a scalar, and then computes the gradient of that scalar, resulting in a vector field
B
Key Concept
Curl and Gradient in Vector Calculus
Explanation
In vector calculus, the curl of a vector field in two dimensions results in a scalar function. Taking the gradient of this scalar function then results in a vector field. Thus, the expression is defined and results in a vector field.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question