
Sia

Question
Math
Posted 10 months ago
Janine is studying the relationship between the size of a diamond (in carats) and its price. She obtains size and price data for a random sample of 64 diamonds. Here is computer output from a least-squares regression analysis on her sample:
\begin{tabular}{lrrrr}
Predictor & Coef & SE Coef & T & P \\
\hline Constant & 8.00 & 60.76 & 0.13 & 0.90 \\
Carat & 2722.34 & 259.92 & 10.47 & 0.00 \\
S & R-sq & & &
\end{tabular}
Assume that all conditions for inference have been met.
Which of these is a confidence interval for the slope of the least squares regression line?
Choose 1 answer:
(A)
(B)
(C)
()
(ㄷ) 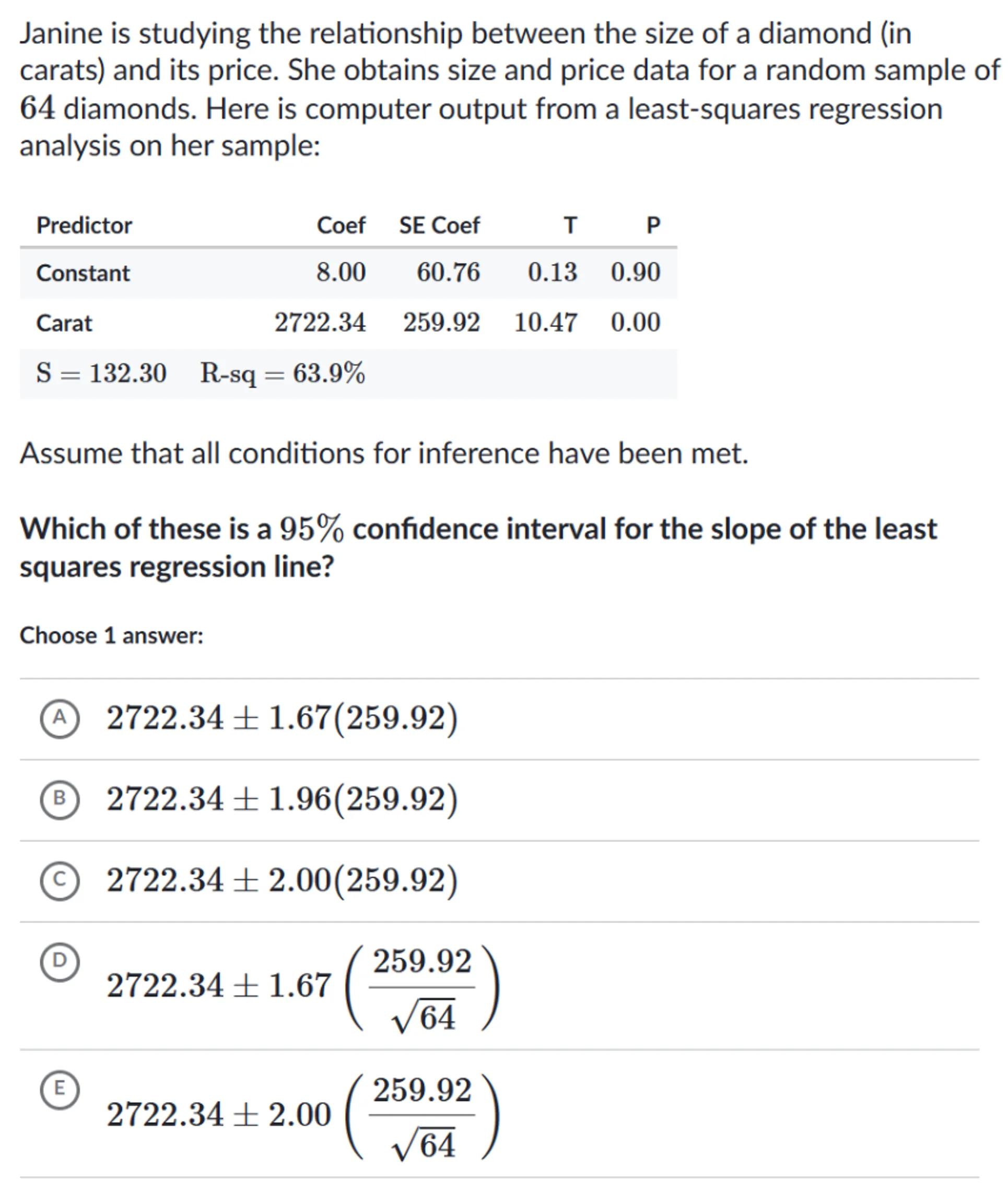

Answer from Sia
Posted 10 months ago
Solution by Steps
step 2
The t-distribution critical value for 95% confidence and 63 degrees of freedom is approximately 2.00 (from asksia-ll calculation list)
step 3
The standard error of the slope (SE Coef) is given as 259.92. Since the sample size is 64, we divide the SE Coef by the square root of the sample size to get the standard error of the estimate of the slope
step 4
Calculating the standard error of the estimate:
step 5
Multiplying the t-distribution critical value by the standard error of the estimate gives us the margin of error for the 95% confidence interval
step 6
Calculating the margin of error:
step 7
The 95% confidence interval for the slope is then
step 8
Substituting the margin of error into the confidence interval formula:
ㄷ
Key Concept
Confidence Interval for Slope
Explanation
The 95% confidence interval for the slope of the least squares regression line is calculated using the t-distribution critical value, the standard error of the slope, and the sample size.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question