
Sia

Question
Math
Posted 10 months ago
In the diagram below, .
Complete the proof that a parallelogram whose diagonals are congruent has a right angle.
This proof uses this theorem: Opposite sides in a parallelogram are congruent.
\begin{tabular}{lll}
& Statement & Reason \\
\hline 1 & and & Given \\
& & \\
2 & & Opposite sides in a parallelogram are congruent. (1) \\
3 & & Line segments are congruent to themselves. \\
4 & & Given \\
5 & Pick statement & Side-side-side congruence (2,3,4) \\
6 & Pick statement & Pick reason \\
7 & & When a transversal crosses parallel lines, same-side \\
interior angles sum up to . (1)
\end{tabular}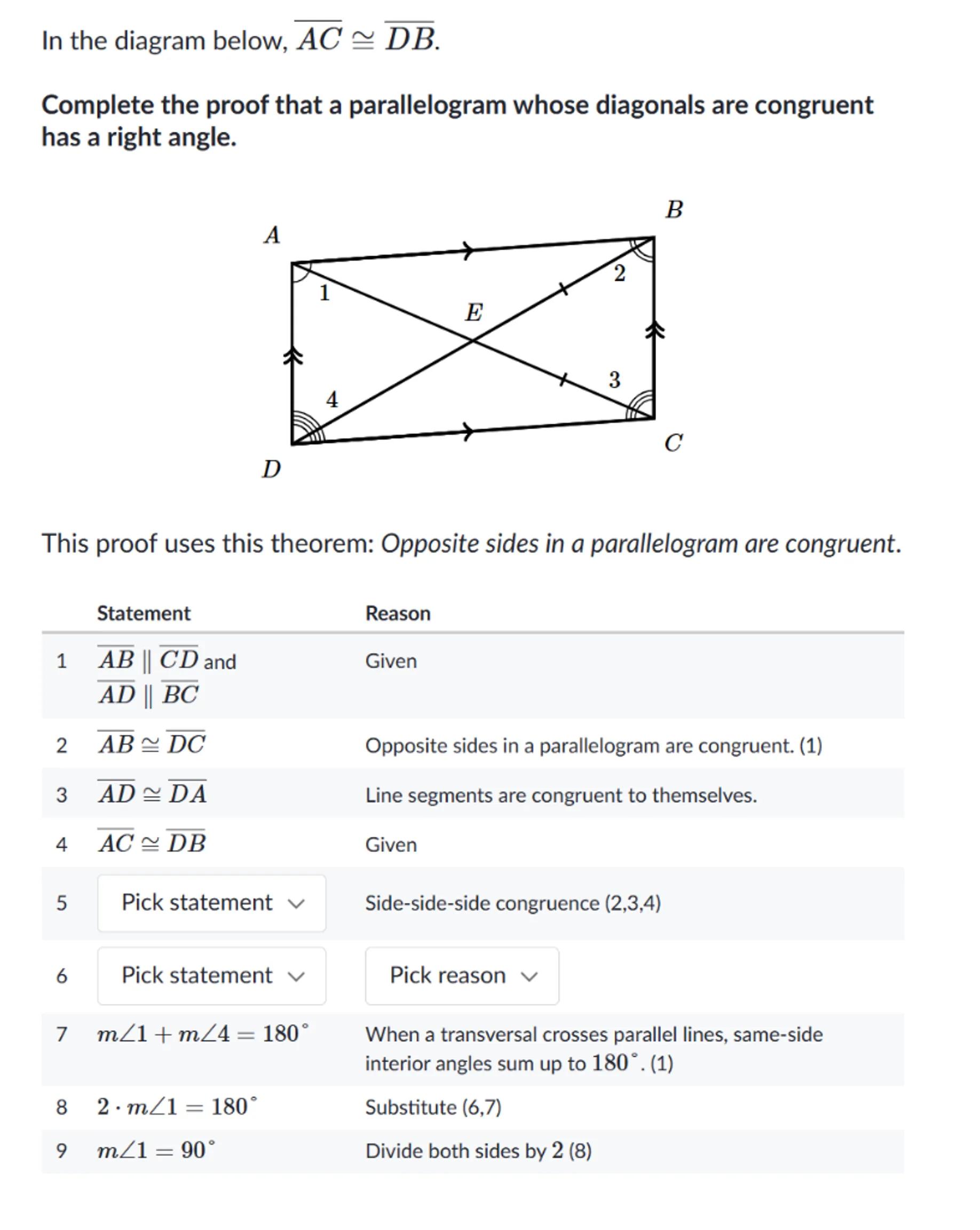

Answer from Sia
Posted 10 months ago
Solution by Steps
step 1
To prove that a parallelogram with congruent diagonals has a right angle, we need to show that the triangles formed by the diagonals are congruent
step 2
By the given information, we know that and (opposite sides of a parallelogram are congruent)
step 3
Since is congruent to itself (reflexive property), we have all three sides of congruent to the corresponding sides of
step 4
By the Side-Side-Side (SSS) congruence postulate,
step 5
From the congruence of the triangles, we know that corresponding angles are congruent, so
step 6
Since and is a transversal, and are same-side interior angles
step 7
By the same-side interior angles theorem, . Since , we can substitute to get
step 8
If two angles sum to and are congruent, each must be . Therefore,
Answer
The parallelogram has a right angle at each vertex, proving that a parallelogram with congruent diagonals is a rectangle.
Key Concept
Side-Side-Side (SSS) Congruence Postulate
Explanation
The SSS Congruence Postulate states that if three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent. This leads to the conclusion that corresponding angles are congruent, and in the case of a parallelogram with congruent diagonals, it results in right angles at each vertex.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question