
Sia

Question
Biology
Posted 9 months ago
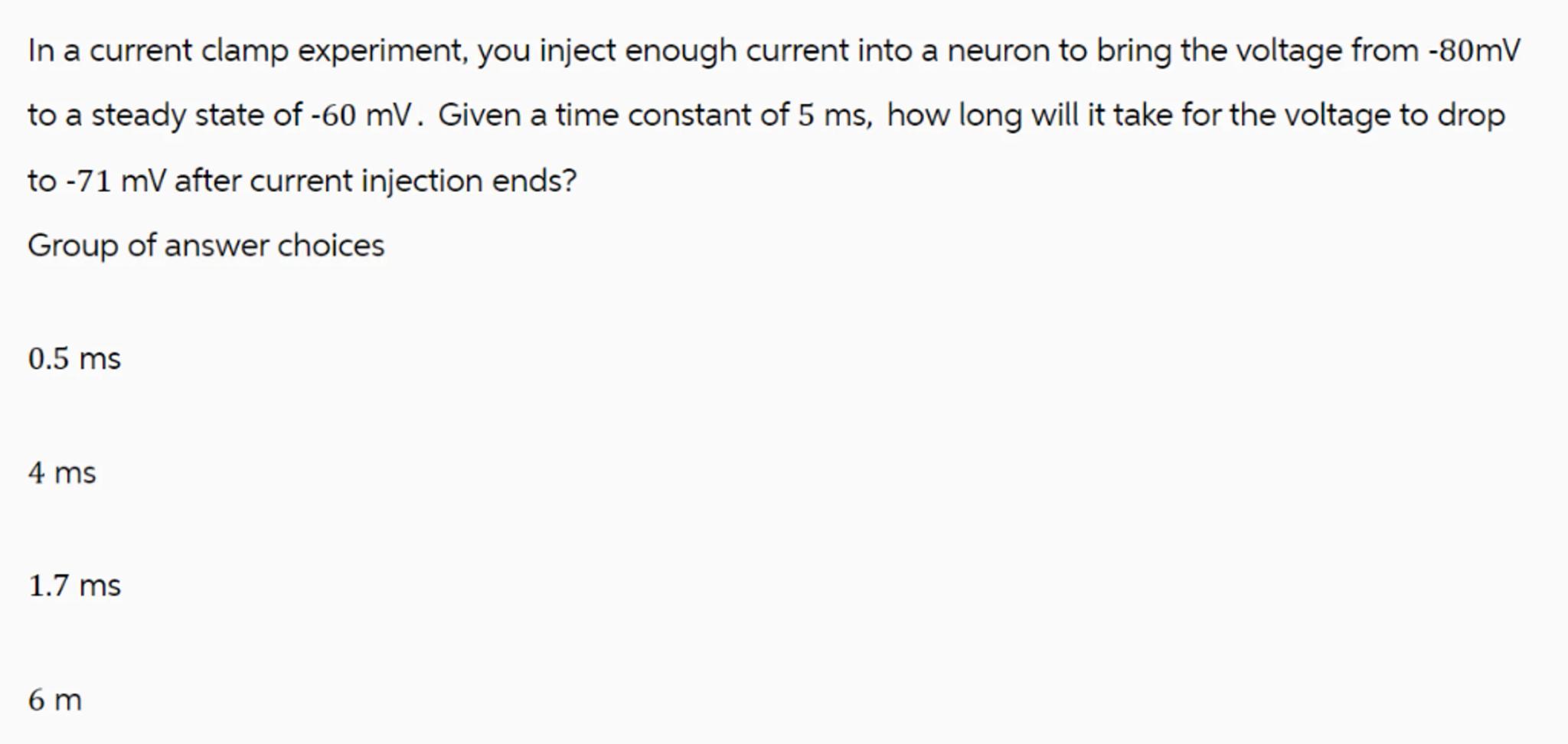
In a current clamp experiment, you inject enough current into a neuron to bring the voltage from to a steady state of . Given a time constant of , how long will it take for the voltage to drop to after current injection ends?
Group of answer choices

Answer from Sia
Posted 9 months ago
B
Key Concept
Voltage decay in a neuron post-current injection
Explanation
The voltage decay after current injection in a neuron can be described by the time constant (τ). The time constant is the time it takes for the voltage to change by 63% of the difference between its initial value and its final value. Since the voltage changes from -60 mV to -71 mV, which is a 63% change towards the resting potential (-80 mV), it takes approximately one time constant, which is 5 ms. However, the question asks for the time to reach -71 mV, which is a 37% change (100% - 63%) towards the resting potential. This corresponds to approximately 0.37 of the time constant. Therefore, 0.37 * 5 ms = 1.85 ms, and the closest answer choice to this calculation is 1.7 ms.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question