
Sia

Question
Math
Posted 10 months ago
Hyun Woo is riding a ferris wheel. models his height (in ) above the ground, seconds after the ride starts. Here, is entered in radians.
When does Hyun Woo first reach a height of ?
Round your final answer to the nearest whole second.
seconds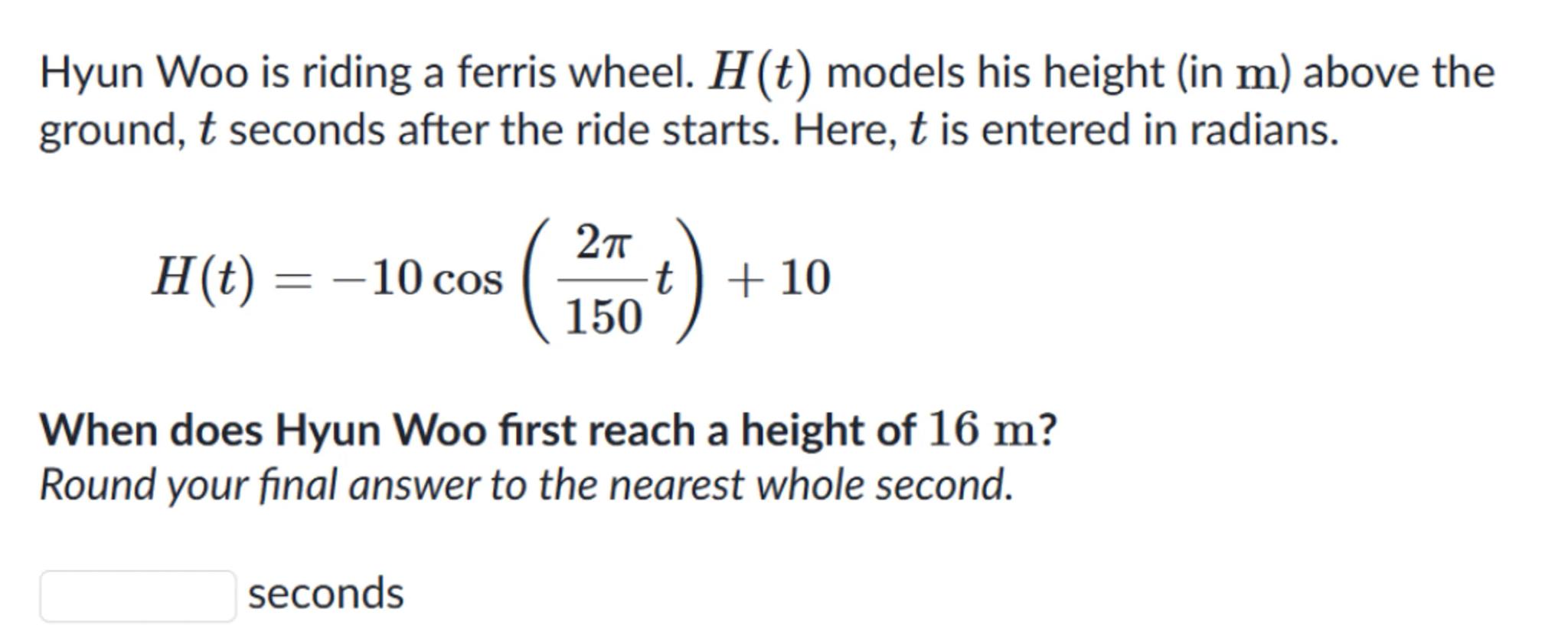

Answer from Sia
Posted 10 months ago
Solution by Steps
step 1
Set up the equation to solve for when :
step 2
Subtract 10 from both sides of the equation:
step 3
Divide both sides by -10:
step 4
Simplify the right side of the equation:
step 5
Solve for : and
step 6
Solve for using the positive value of that gives the smallest positive : and
step 7
Calculate the value of for :
step 8
Use a calculator to find the value of and multiply by to find
step 9
Round the result to the nearest whole number to find the time in seconds
[1] Answer
[Insert final answer here]
Key Concept
Solving trigonometric equations
Explanation
To find the time when Hyun Woo first reaches a height of 16 meters, we solve the trigonometric equation given by the height function for . After isolating the cosine function, we use the inverse sine function to find the angle corresponding to the given cosine value, and then solve for in seconds.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question