
Sia

Question
Math
Posted 10 months ago
Evaluate the iterated integral.
Choose 1 answer:
(A)
(B)
(C)
(D) 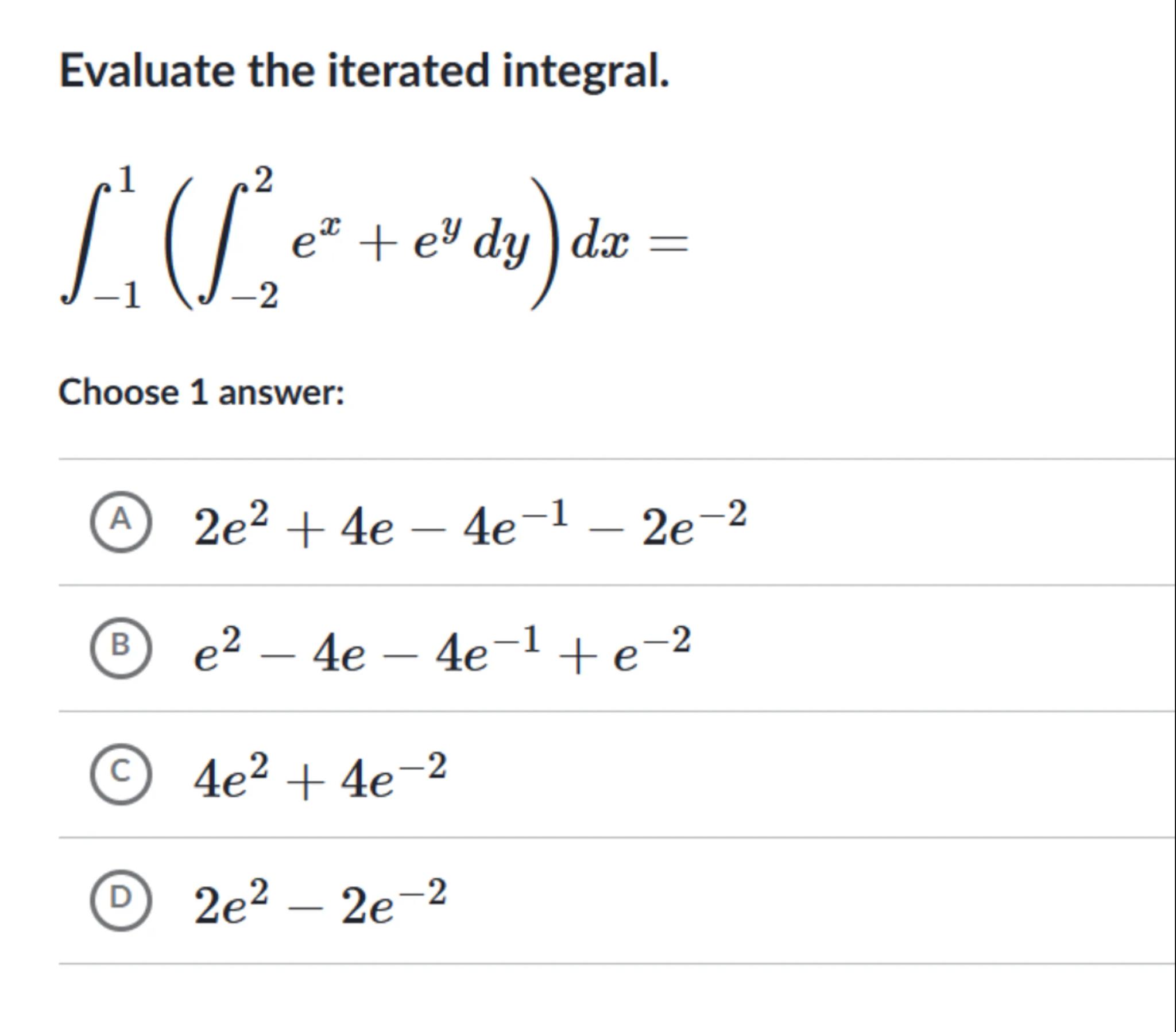

Answer from Sia
Posted 10 months ago
Solution by Steps
step 2
Since is a constant with respect to , the integral becomes
step 3
Compute the integrals:
step 4
Substitute the limits of integration:
step 5
Simplify the expression:
step 6
Evaluate the outer integral with respect to :
step 7
Split the integral:
step 8
Since is constant with respect to , the second integral becomes
step 9
Compute the integrals:
step 10
Substitute the limits of integration:
step 11
Simplify the expression:
step 12
Factor out common terms:
step 13
Combine like terms:
A
Key Concept
Iterated Integration
Explanation
When evaluating an iterated integral, we integrate with respect to one variable at a time while treating the other variable as a constant. After integrating with respect to the inner variable, we substitute the limits of integration for that variable, and then integrate the resulting expression with respect to the outer variable.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question