
Sia

Question
Math
Posted 9 months ago
Consider the following problem:
The horizontal leg of a right triangle is increasing at a rate of and the vertical leg of the triangle is increasing at a rate of . At a certain instant , the horizontal leg is and the vertical leg is . What is the rate of change of the length of the hypotenuse at that instant?
Match each expression with its units.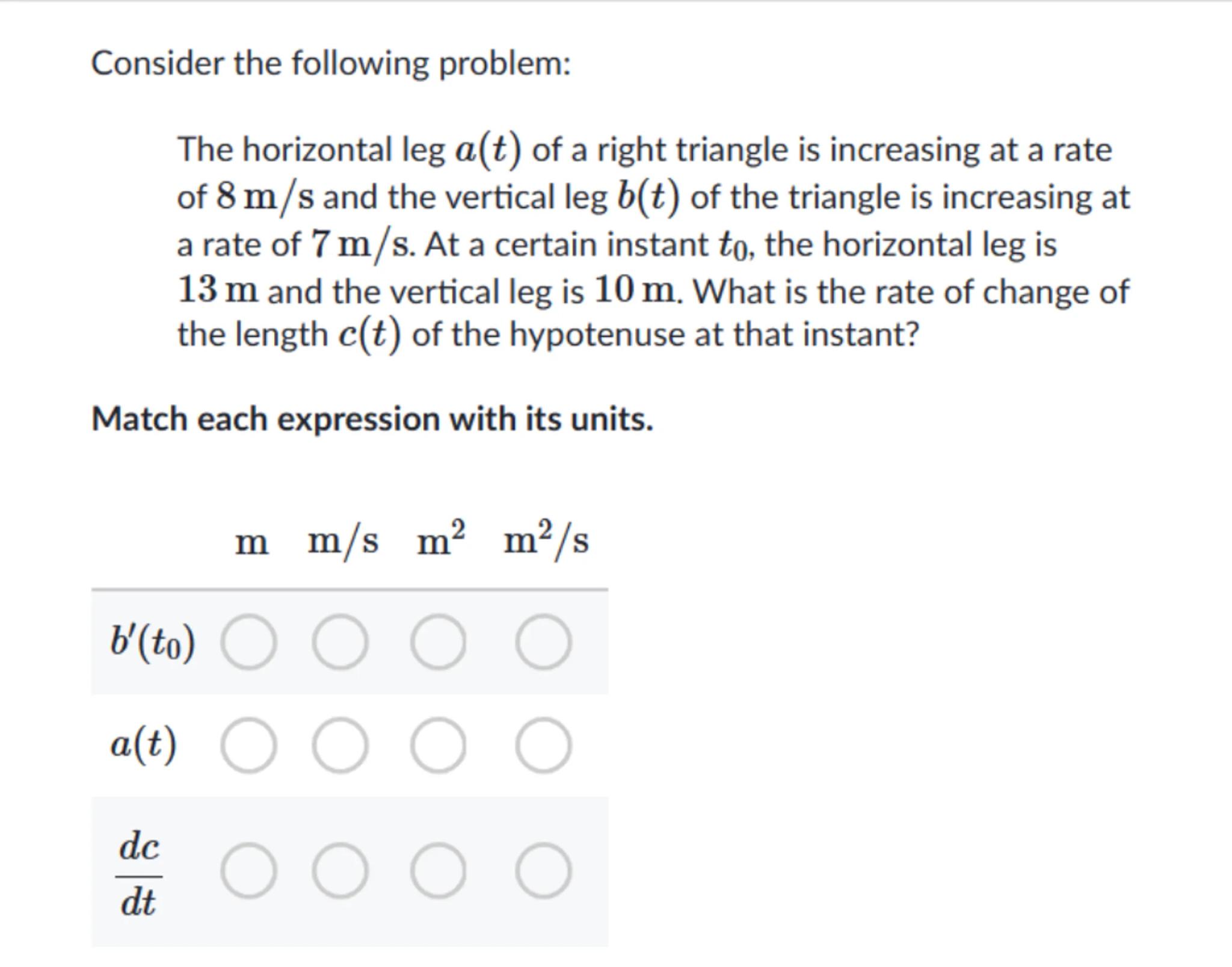

Answer from Sia
Posted 9 months ago
Solution by Steps
step 1
We start by noting the given information: , , , and . We need to find , where is the hypotenuse of the right triangle
step 2
Using the Pythagorean theorem, we have
step 3
Differentiating both sides with respect to , we get
step 4
Applying the chain rule, we obtain
step 5
Simplifying, we get
step 6
Substituting the given values, we have
step 7
Simplifying further,
Answer
The rate of change of the length of the hypotenuse is approximately .
Key Concept
Rate of change of the hypotenuse in a right triangle
Explanation
The rate of change of the hypotenuse can be found by differentiating the Pythagorean theorem with respect to time and substituting the given rates of change of the legs.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question