
Sia

Question
Statistics
Posted 8 months ago
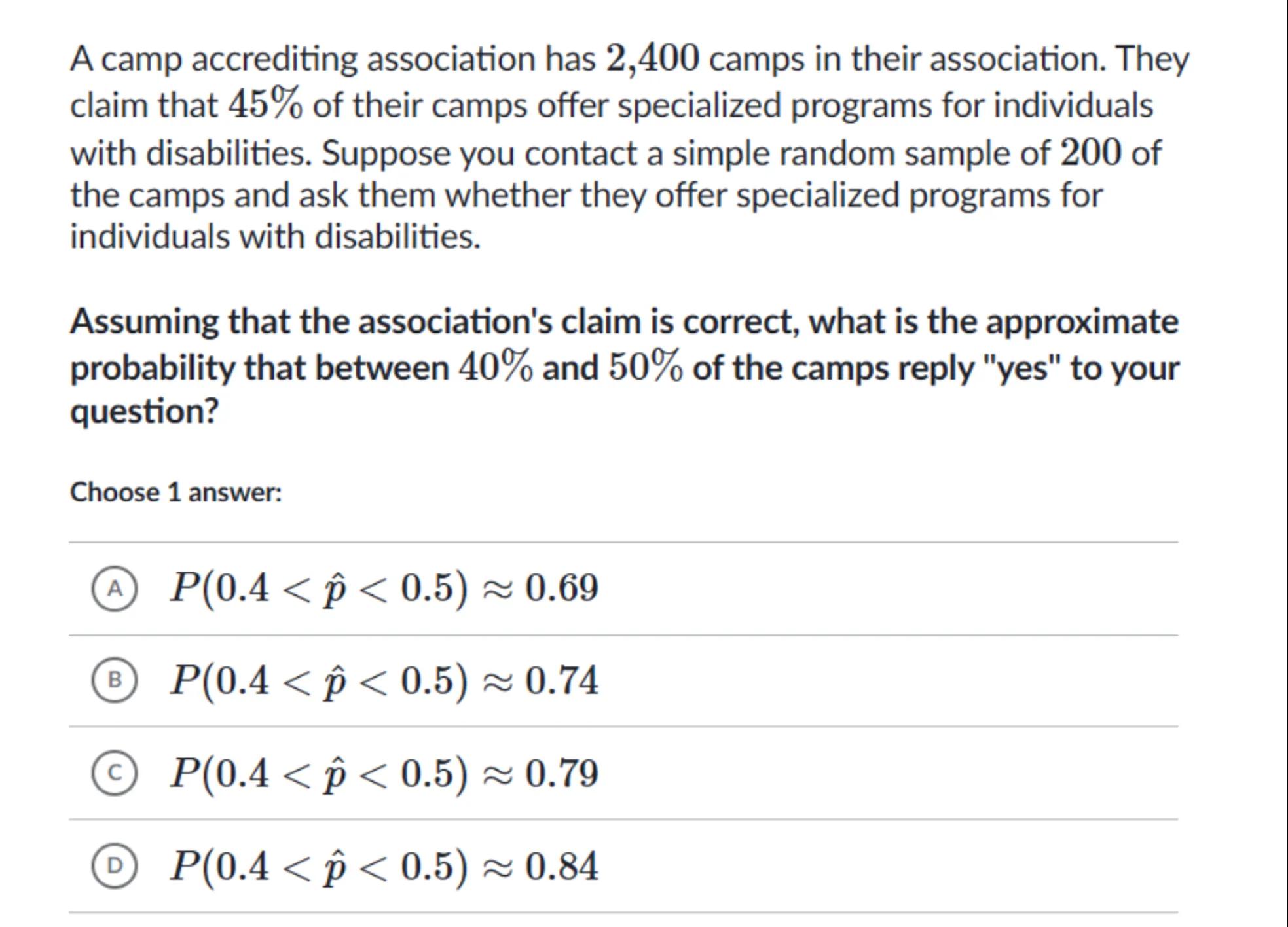
A camp accrediting association has 2,400 camps in their association. They claim that of their camps offer specialized programs for individuals with disabilities. Suppose you contact a simple random sample of 200 of the camps and ask them whether they offer specialized programs for individuals with disabilities.
Assuming that the association's claim is correct, what is the approximate probability that between and of the camps reply "yes" to your question?
Choose 1 answer:
(A)
(B)
(c)
() 

Answer from Sia
Posted 8 months ago
Solution by Steps
step 1
Identify the given parameters: The population proportion , the sample size , and the range of interest
step 2
Calculate the standard error (SE) of the sample proportion using the formula: . Substituting the values, we get
step 3
Convert the range of sample proportions to z-scores using the formula: . For , . For ,
step 4
Use the standard normal distribution table to find the probabilities corresponding to the z-scores. and
step 5
Calculate the probability that the sample proportion is between 0.4 and 0.5:
Answer
D
Key Concept
Standard Error and Z-scores
Explanation
The standard error measures the variability of the sample proportion, and z-scores help convert sample proportions to standard normal distribution values to find probabilities.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question