
Sia
24. (T/F) Not every orthogonal set in is linearly independent.
25. (T/F) If is a linear combination of nonzero vectors from an orthogonal set, then the weights in the linear combination can be computed without row operations on a matrix.
26. (T/F) If a set has the property that whenever , then is an orthonormal set.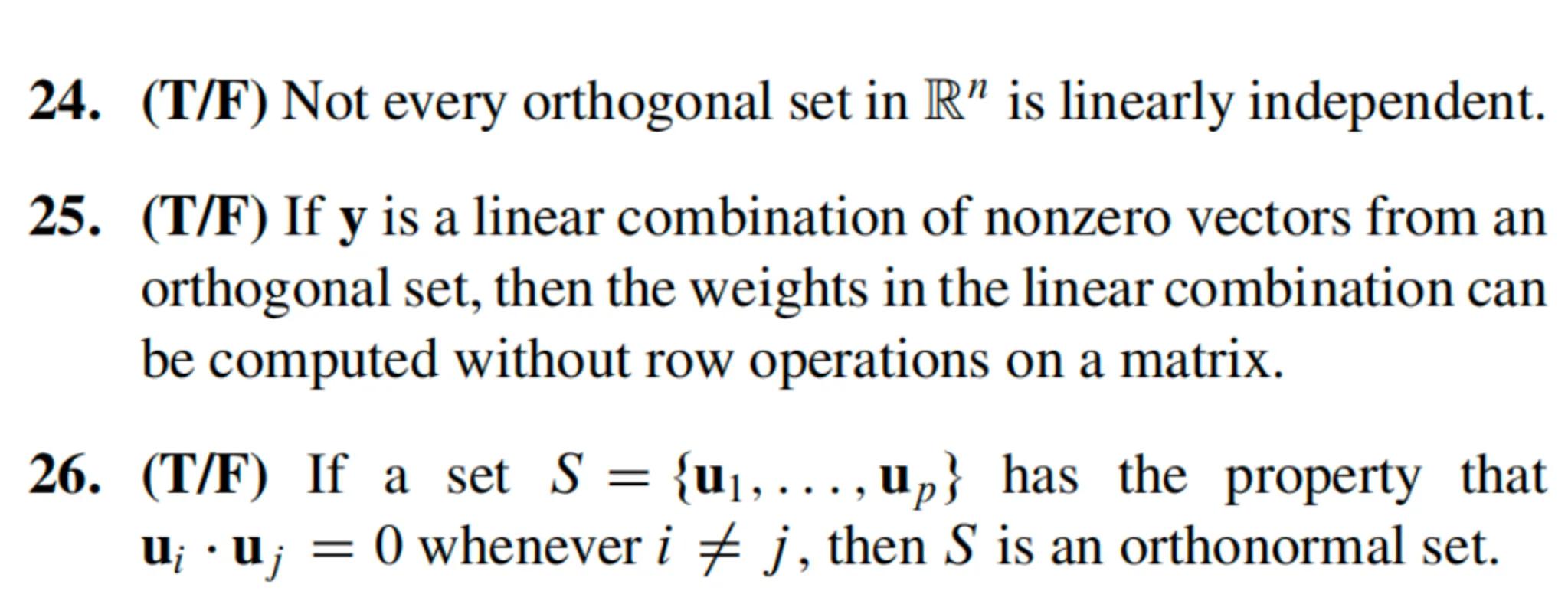

Answer from Sia
Posted 9 months ago
Solution by Steps
step 1
Identify the statement: "Not every orthogonal set in is linearly independent."
step 2
Recall the definition of an orthogonal set: A set of vectors is orthogonal if for all
step 3
Recall the property of orthogonal sets: In , any orthogonal set of nonzero vectors is linearly independent
step 4
Conclude that the statement is False because every orthogonal set of nonzero vectors in is linearly independent
Answer
False
Key Concept
Orthogonal sets and linear independence
Explanation
In , any orthogonal set of nonzero vectors is always linearly independent.
Question 25
step 1
Identify the statement: "If is a linear combination of nonzero vectors from an orthogonal set, then the weights in the linear combination can be computed without row operations on a matrix."
step 2
Recall the property of orthogonal sets: If is an orthogonal set, then any vector that is a linear combination of these vectors can be written as
step 3
The weights can be computed using the formula , which does not require row operations on a matrix
step 4
Conclude that the statement is True because the weights can be computed directly using the dot product
Answer
True
Key Concept
Weights in linear combinations of orthogonal sets
Explanation
The weights in the linear combination of vectors from an orthogonal set can be computed directly using dot products, without the need for row operations on a matrix.
Question 26
step 1
Identify the statement: "If a set has the property that whenever , then is an orthonormal set."
step 2
Recall the definition of an orthonormal set: A set of vectors is orthonormal if for all and for all
step 3
Note that the given property for only ensures orthogonality, not orthonormality
step 4
Conclude that the statement is False because orthonormality also requires each vector to have a unit norm, which is not guaranteed by orthogonality alone
Answer
False
Key Concept
Orthogonal vs. Orthonormal sets
Explanation
An orthonormal set requires both orthogonality and unit norm for each vector, whereas the given property only ensures orthogonality.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question