
Sia
The second difference of question c is constant
2. Each of the following tables shows a collection of points from a function . For each table, determine whether the is possibly linear, possibly exponential, or cannot be either.
If you decide that is exponential or linear, provide the rule for the function.
(a)
\begin{tabular}{|l|l|l|l|l|l|}
\hline & -1 & 0 & 1 & 2 & 3 \\
\hline & 5 & 2 & -1 & -4 & -7 \\
\hline
\end{tabular}
(b)
\begin{tabular}{|l|l|l|l|l|l|}
\hline & -1 & 0 & 1 & 2 & 3 \\
\hline & 32 & 16 & 8 & 4 & 2 \\
\hline
\end{tabular}
(c)
\begin{tabular}{|l|l|l|l|l|l|}
\hline & -1 & 0 & 1 & 2 & 3 \\
\hline & 2 & 4 & 7 & 11 & 16 \\
\hline
\end{tabular}
[3 marks]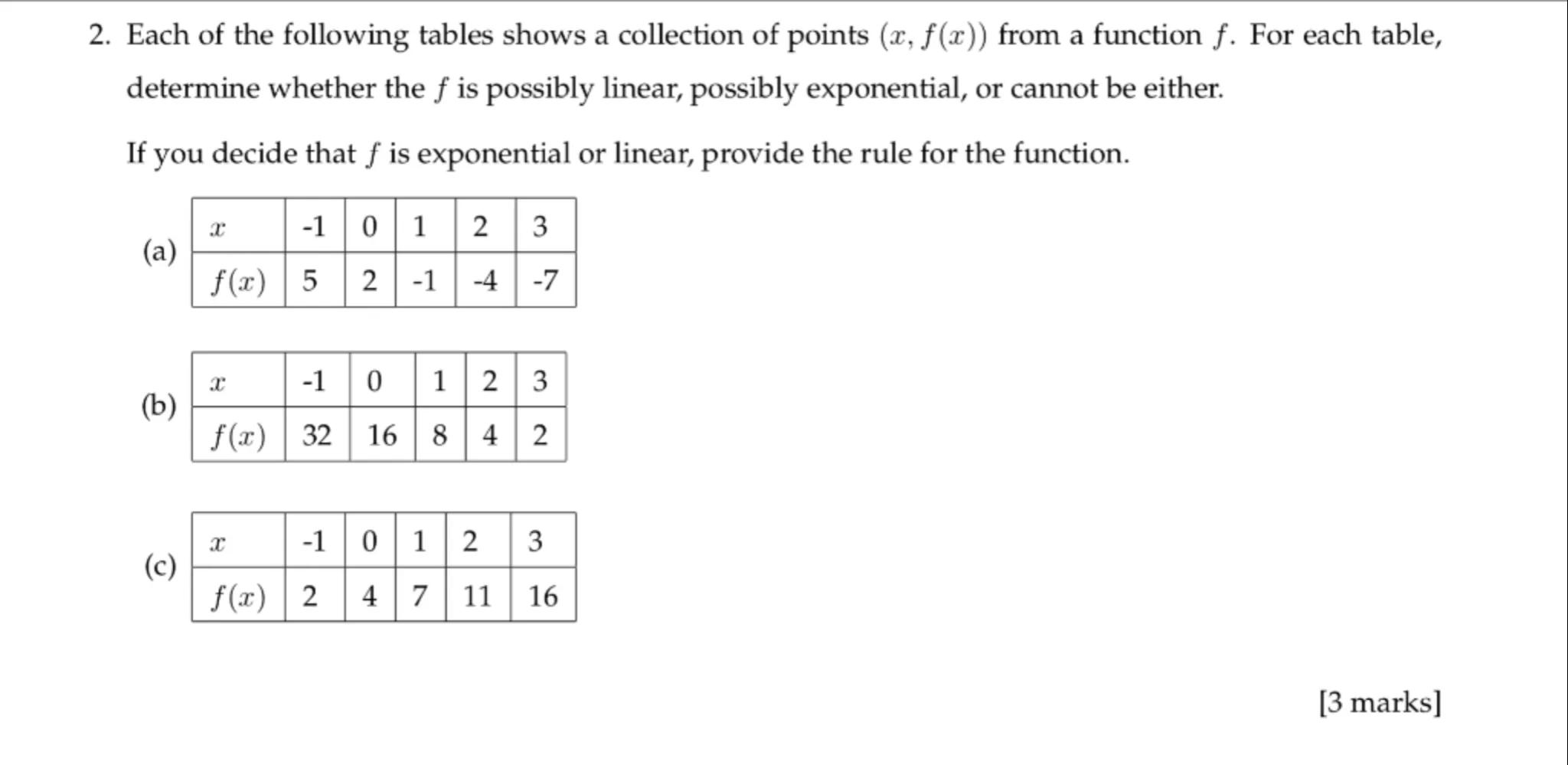

Answer from Sia
Posted 4 months ago
Solution by Steps
step 1
For table (a), we observe the values of : 5, 2, -1, -4, -7. The differences between consecutive values are: , , , and . Since the first differences are constant, the function is possibly linear
step 2
The linear function can be expressed in the form . Using the points (0, 2) and the slope , we can find the equation. The equation becomes
step 3
For table (b), the values of are: 32, 16, 8, 4, 2. The ratios of consecutive values are: , , , and . Since the ratios are constant, the function is possibly exponential
step 4
The exponential function can be expressed in the form . Using the point (0, 32) and the ratio , we find
step 5
For table (c), the values of are: 2, 4, 7, 11, 16. The differences between consecutive values are: , , , and . The differences are not constant, and the second differences are also not constant, indicating that the function is neither linear nor exponential
Answer
(a) Linear: ; (b) Exponential: ; (c) Neither
Key Concept
Identifying function types based on data points
Explanation
By analyzing the differences and ratios of the function values, we can determine if the function is linear, exponential, or neither, and derive the corresponding equations when applicable.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question