
Sia
1. The decomposition of ethanol on an alumina surface
was studied at . Concentration versus time data were collected for this reaction, and a plot of versus time resulted in a straight line with a slope of .
a) Determine the rate law, the integrated rate law, and the value of the rate constant for this reaction.
b) If the initial concentration of was , calculate the half-life for this reaction.
c) How much time is required for all the to decompose?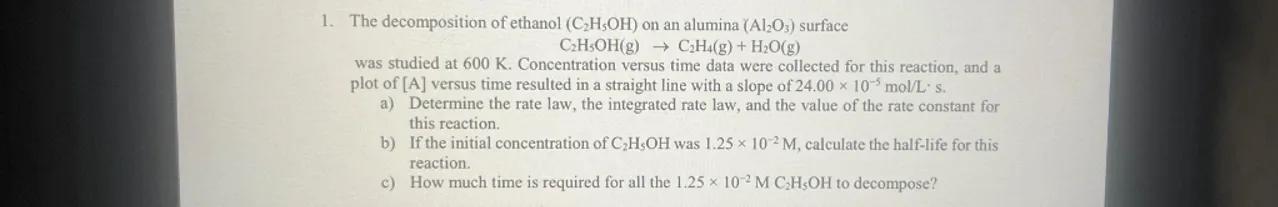

Answer from Sia
Posted 10 months ago
Solution
1
Determine the order of the reaction: Since a plot of versus time yields a straight line, the reaction is first-order
2
Write the rate law: For a first-order reaction, the rate law is , where is the rate constant and is the concentration of the reactant
3
Calculate the rate constant \(k\): The slope of the concentration versus time plot for a first-order reaction is equal to . Thus,
4
Write the integrated rate law: For a first-order reaction, the integrated rate law is , where is the initial concentration
5
Calculate the half-life (\(t_{1/2}\)): The half-life for a first-order reaction is given by . Substituting the value of gives
6
Determine the time for complete decomposition: For a first-order reaction, the time required for complete decomposition is theoretically infinite. However, we can calculate the time for the concentration to decrease to a negligible amount, such as of the initial concentration
1 Answer
Rate law:
Integrated rate law:
Rate constant:
2 Answer
Half-life:
3 Answer
Time for complete decomposition: Theoretically infinite, but can calculate time to reach a negligible concentration.
Key Concept
The slope of the concentration versus time plot for a first-order reaction is equal to the negative of the rate constant.
Explanation
Since the plot of versus time is linear, the reaction follows first-order kinetics, and the slope gives the rate constant.
Key Concept
The half-life of a first-order reaction is independent of the initial concentration and is calculated using the rate constant.
Explanation
The half-life for a first-order reaction is given by , which only depends on the rate constant.
Key Concept
Complete decomposition in a first-order reaction takes an infinite amount of time, but practical completion can be calculated.
Explanation
Although complete decomposition is never reached, we can determine the time required for the concentration to fall to a very low level, such as of the initial
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question